By Vincent Pica, Commodore, First District, Southern Region (D1SR), United States Coast Guard Auxiliary
Going back centuries, journals of seafarers are peppered with language indicating that they knew the Earth was round. “In the offing” meant, and means today, the waters you can see from where you are to the horizon. “Ahoy, captain, vessel off the starboard bow! Hull down, sir,” might yell the lookout from the crow’s nest aloft. This meant that all he could see from his vantage point were the sails – the ship’s hull was still below the horizon. So, “round has been around” (pun intended) for thousands of years. How many thousands? About 22 centuries before the epic confrontation between Galileo and the medieval Church, Phoenician sailors circumnavigated Africa, sailing down the east coast and back up the western shores, through the “Pillars of Hercules” at Gibraltar and back to Egypt, to report to the Pharaoh that, indeed, the world must be round.
Why? Because, once they crossed southbound what we now know as the equator, the sun had “flipped around” to reside on the opposite side of their sailing vessel on a given tack. At night, the moon would have become “left-handed” rather than “right-handed*” as we see it at night. Thus, they knew the world must round, for, if it were flat, such observations couldn’t occur. So, mirabile dictu, as the Romans would come to say, the world was round – but no one knew how big it was.
It took a Greek, named Eratosthenes, in 240 BC to figure out how big the Earth was. He noticed that in Aswan, a place then called Syene, around the time of the summer solstice, the sun shined directly to the bottom of a well and nearby posts threw no shadows on the ground. To do that, the sun had to be directly overhead. At the same time, where he lived in Alexandria to the north, a post would throw a shadow onI the ground. For both those conditions to be true, the Earth had to be round and the rest, i.e., how big around was it, was simply some algebra and geometry which, as every high school student knows, the Greeks were very good at. So, Q.E.D, this column is about that math and thus starts a series on celestial navigation.
Upon One Principle, Celestial Navigation Rests
But what did ol’ Eratosthenes do? Well, the shadow from the post in Alexandria created an angle. Having that in hand, plus the “arc distance” from Syene to Alexandria, we have the beginnings of a formula. This angle created by the shadow of the post in Alexandria, called “theta” to this day and displayed as 0, is exactly the same angle that would be found at the center of the Earth, if the sunlight shining down that well in Syene could reach the center of the Earth and then “bounce” back up and out through Alexandria. In plain speak, the angle from Syene down to the center of the Earth and back out through Alexandria is exactly the same angle as that created by the sun’s shadow thrown from the post in Alexandria. See diagram.
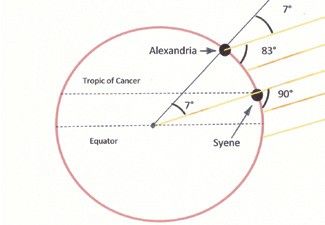
Thus, Eratosthenes deduced that the arc distance (d) is proportional to the angle 0. If you halve the angle, you halve the distance. This means that you can create a formula from these facts: d = constant x 0. Eratosthenes realized further that “d” would be equal to the circumference of the Earth when the angle, 0, was 360°.
D = (circumference/360°) x 0 had to be the circumference when 0 was 360°!
Since this was true, all he needed to do was figure out what the constant was, which, knowing the distance from Syene to Alexandria being about 500 miles, he did some algebra and solved for the circumference of the Earth.
Eratosthenes solved the equation above and determined the Earth’s circumference to be 25,500 miles. With our advanced technology, we know its circumference to be 24,874 miles…pretty darn good for ol’ Eratosthenes with 240 BC technology!
So, upon exactly what principle does celestial navigation rest? Eratosthenes’ formula enables someone to determine how far they are from someplace else. If we take Eratosthenes’ formula one step further, i.e., to solve for the constant that, when multiplied by the observed angle as measured at noon, tells you how far away you are from the “well”, i.e., Greenwich, England, we get this:
D = (circumference/360°) x 0
We substitute ‘circumference’ for its equivalent – 2*pi*R (where R is the Radius of the Earth, which we know today to be 3,440 nautical miles)
D = (2*pi*3440)/ 360°) x 0
D = 60.04 x 0 or, rounding off,
D = 60nm
That is the constant and why 60 nautical miles is equal to 1 degree of latitude. This is the kernel upon which celestial navigation rests!
If you are interested in being part of the USCG Forces, email me at JoinUSCGAux@aol.com or go direct to the D1SR Human Resources department, who are in charge of new members matters, at
d1south.org/StaffPages/DSO-HR.php and we will help you “get in this thing.”
* Being “right-handed” means that the Earth’s shadow moves from the right side of the moon to the left as the moon wanes from a full moon to a new moon, as viewed from the northern hemisphere.
Captain Kevin Reed is the Captain of the Port and Sector Commander for U.S. Coast Guard Sector Long Island Sound. CAPT Reed is responsible for all active-duty, reservist and auxiliary Coast Guard personnel within the Sector. As a Commodore of the U.S. Coast Guard Auxiliary First District, Southern Region, Vin Pica works closely with CAPT Reed and his staff to promote boating safety in the waters between Connecticut, Long Island and 200 nautical miles offshore. Sector Long Island Sound Command Center can be reached 24 hours a day at 203-468-4401.



